
Revetment Design
Redesigning the revetment at Alpha Iota Lodge involves several factors. The type of rock used, size of rock used, type of revetment, and dimensions of the revetment structure are all dependent on the expected wave conditions. To determine the expected wave conditions the JONSWAP and CERC-Weggel method were used. These methods look at expected maximum wind speeds and calculate the wave heights that would correspond with this wind speed using assumptions about wave height – wind speed relationships and the bathymetry of Lake Mendota.
The maximum mean annual peak wind speed on Lake Mendota was used for the revetment design so that the design is robust for all conditions. The wind speeds used were measured by the Wisconsin State Climatology Office from 1971 until 1993. Using this maximum expected wind speed the significant wave height can be calculated.
Looking at waves as the meet the shore requires the consideration of breaking waves. The CERC-Weggel method, in conjunction with bathymetry data from the Wisconsin Department of Natural Resources, was used to determine the maximum breaking wave height, which was used as the maximum wave height for the revetment design.
Using the maximum expected wave height at shore, the various parts of the revetment design were calculated using Chapter 16 of the Wisconsin Supplement Engineering Field Handbook. This chapter lays out state approved methods for streambank and shoreline protection in Wisconsin. Using the handbook, the revetment dimensions and rock needed for a robust design were found.
Revetment Results
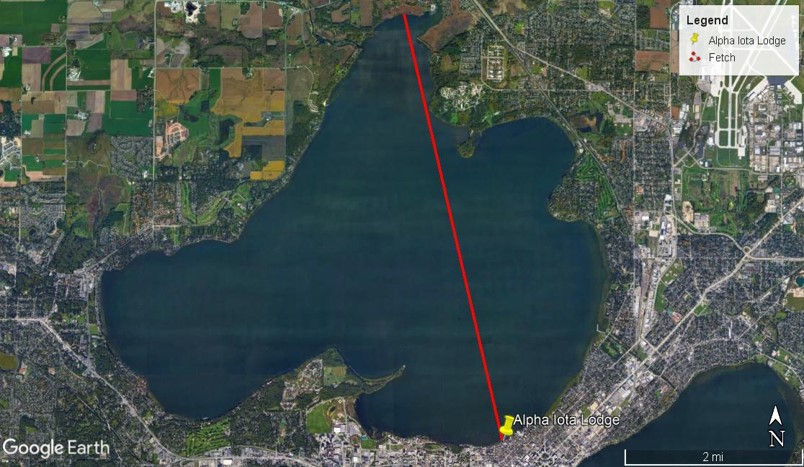
The maximum uninterrupted distance wind can travel across Lake Mendota to Alpha Iota is required to calculate the significant wave properties using the the JONSWAP method. This distance across the lake is called the fetch (F), which was determined using Google Earth, as shown below in Figure 1.
F = 4.84 miles = 7800 meters
Figure 1. The fetch at Alpha Iota was determined with Google Earth.
In addition to the fetch, the maximum wind speed at 10 meters (U10) at the site is also necessary to perform the JONSWAP method. The maximum wind speed was approximated using the maximum values of the Mean Annual Peak Wind Speed on Lake Mendota from the Wisconsin State Climatology Office.
The JONSWAP method uses the fetch (F), expected maximum wind speed at 10 meters (U10), length of storm (td) and acceleration due to gravity (g) to find the significant wave height and period. The length of storm was assumed to be 2 hours (7200 seconds) and the acceleration due to gravity was assumed to be 9.81 meters per second squared. The first step in the JONSWAP method is determining if the waves will be limited by the fetch or the duration of the storm. To be conservative the assumed storm length was chosen so wave conditions would be limited by the fetch distance. An example calculation to determine this assumption was consistent is shown below.

Once the limiting factor in the size of waves is confirmed to be the fetch, a significant wave height (Hs) of 1.25 meters and a period (Tp) of 3.745 seconds was determined. These values are important for producing a thoughtful revetment design.
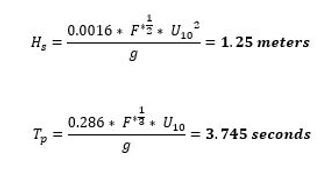
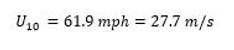
JONSWAP
CERC - Weggel
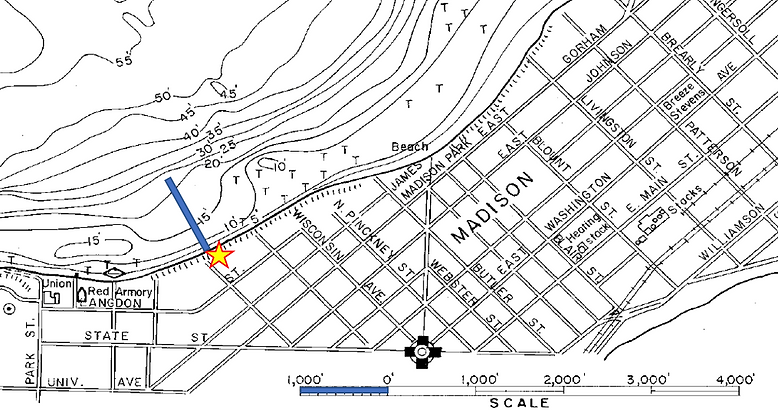
The nearshore slope (m) approaching Alpha Iota was estimated by using a bathymetric contour map of Lake Mendota, which was made available by the Wisconsin Department of Natural Resources (see Figure 2). The slope will cause a runup of the wave and possibly lead to a breaking wave. To accurately design the revetment at Alpha Iota Lodge, the larger of the breaking wave and the significant wave needs to be used.
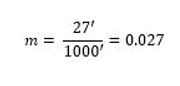
Figure 2. Lake Mendota bathymetric contour map [7].
Using the slope and relationships between Ho (significant wave height, Hs), the wave period (T), and acceleration due to gravity (g), the breaking wave height (Hb) could be calculated.

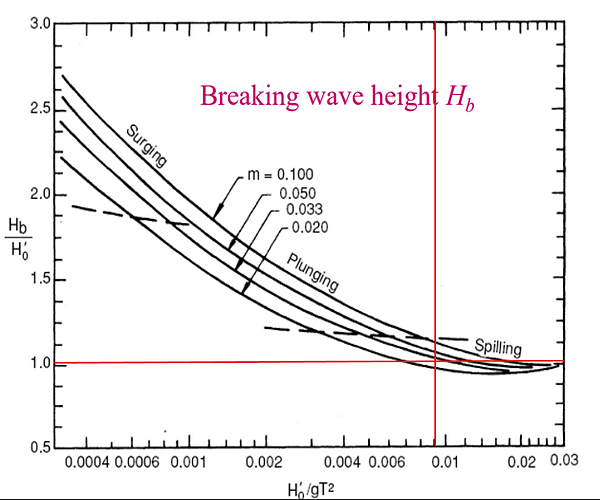
Using the breaking wave graph (see Figure 3) and a slope of 0.027 a relationship between the significant wave height (Hs) and the breaking wave height (Hb) could be found.
Figure 3. Breaking wave height trends with differing slopes (m). Ho' denotes significant wave height (Hs).

Since the breaking wave height is greater than the significant wave height it will be used in the revetment design.
The relationship between wave height and wave length is calculated using the dispersion formula. The wave length is used to calculate the expected runup of the wave, or the vertical distance it will travel up the revetment slope. A water depth (d) was measured at the shore of Alpha Iota Lodge. The wave period (T) was found using the JONSWAP method.
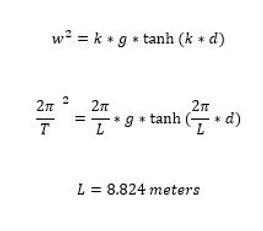
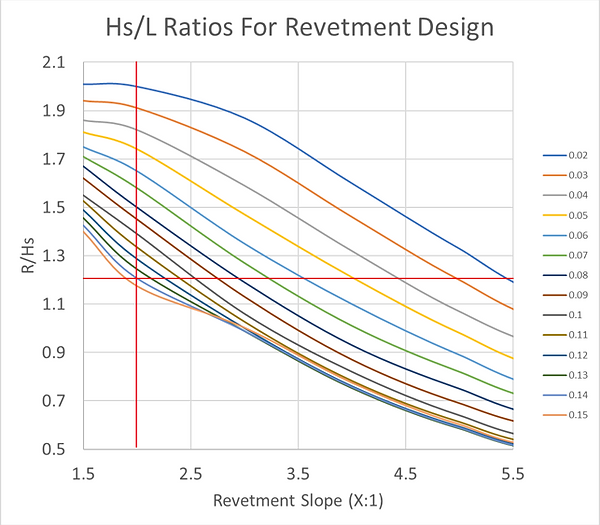
The runup and setup for a revetment design slope of 2:1, or two meters of horizontal distance to one meter of vertical rise, was calculated with the design wave heights and wave lengths. The Ho/L ratio and designed slope are used to find the R/Ho ratio (see Figure 4), and in turn, the runup (R).
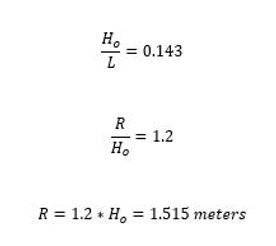
Figure 4. Revetment slope and runup/wave height relationship for different nearshore slopes (slopes shown in legend on the right).
The setup (S) is the vertical rise in water level due to the wind. The Wisconsin Supplement Engineering Field Handbook outlines a piece wise function for the setup, limiting the setup to a maximum of 0.5 feet. The setup for the Alpha Iota Lodge is less than 0.5 feet so the setup formula is valid.
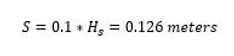
From the runup, setup, and breaking wave height the upper and lower limits of protection can be calculated. These limits of protection are the extents to which revetment must extend above and below the water level to be most effective. Extending revetment below the water level supports the revetment toe and prevents undercutting of the shoreline. The upper limit of the revetment is designed to prevent the waves from overtopping the shore and eroding the toe of the slope.
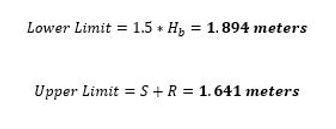
In addition to the upper and lower limits of revetment protection, the appropriate size and type of rock needs to be determined. The mean weight (W50) and mean diameter (D50) of the rock was calculated using strategies laid out in the Wisconsin Supplement Engineering Field Handbook. A rock factor (Factorrock) indicates the angularity of the revetment needed. To reduce the weight of the rock needed an angular rock was selected, indicated by Factorrock = 19.5. A typical rock specific gravity (Gs) of 2.65 was used for the design. The slope of the revetment (Z) is 2:1, indicated by Z=2. A safety factor is included in the formula, however since the wave height used for the revetment design is the significant wave height the safety factor will be 1. Therefore, Hrock = H = 1.2625 meters.

The rock selected for the revetment will follow the gradation curve (see Figure 5) created using the EFH 17 method. The EFH 17 gradation method for revetment gives a well graded aggregate selection. A well graded aggregate will reduce leeching from the slope into Lake Mendota as well as increase the strength of the revetment.
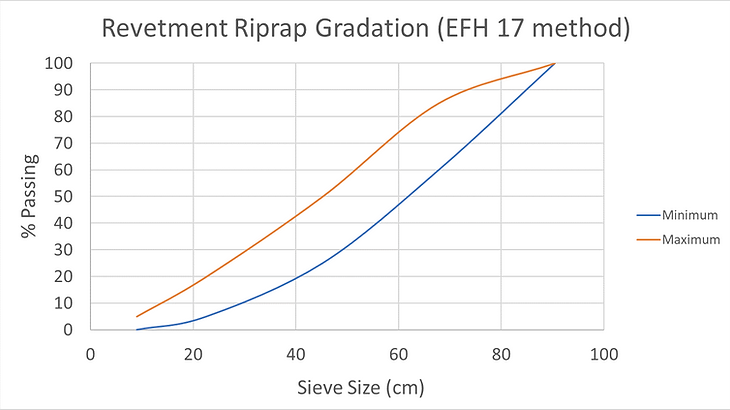
Figure 5. Revetment riprap gradation curve.
